Click here and press the right key for the next slide (or swipe left)
also ...
Press the left key to go backwards (or swipe right)
Press n to toggle whether notes are shown (or add '?notes' to the url before the #)
Press m or double tap to slide thumbnails (menu)
Press ? at any time to show the keyboard shortcuts

How to Distinguish Two (Or More) Systems for Social Cognition
[email protected]

system ?!
Are ‘the dichotomous characteristics used to define the two-system models … perfectly correlated’?
[and] whether a hybrid system that combines characteristics from both systems could not be … viable’
Keren and Schul (2009, p. 537)
‘the process architecture of social cognition is still very much in need of a detailed theory’
Adolphs (2010 p. 759)
case study 1
speech perception
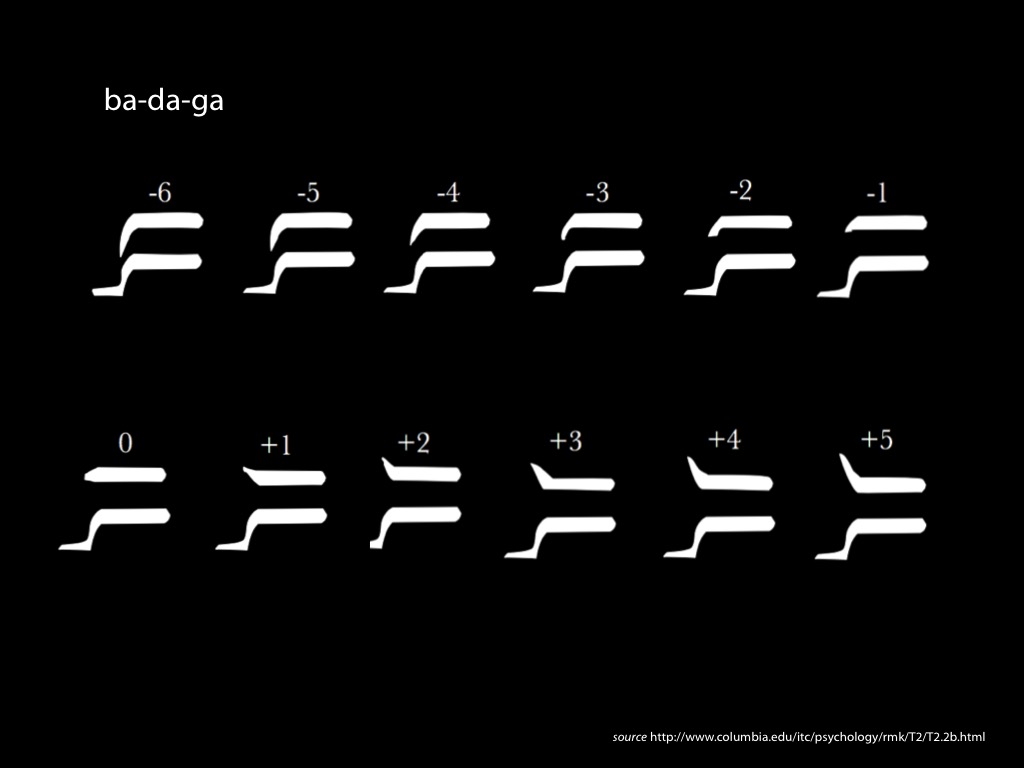
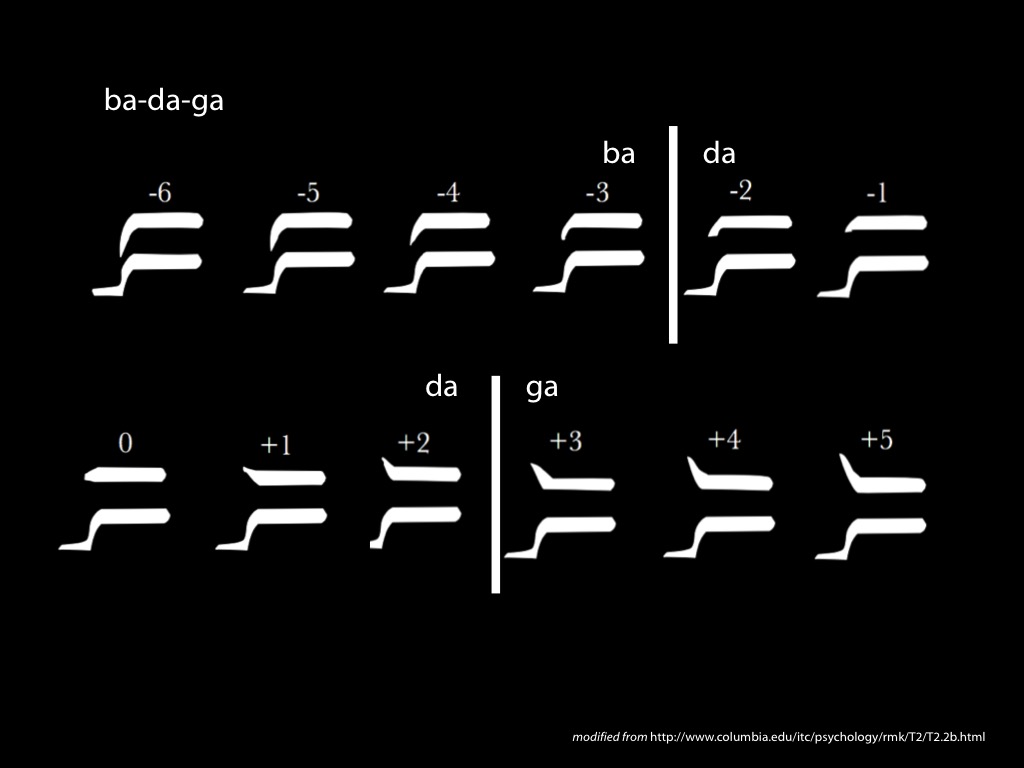

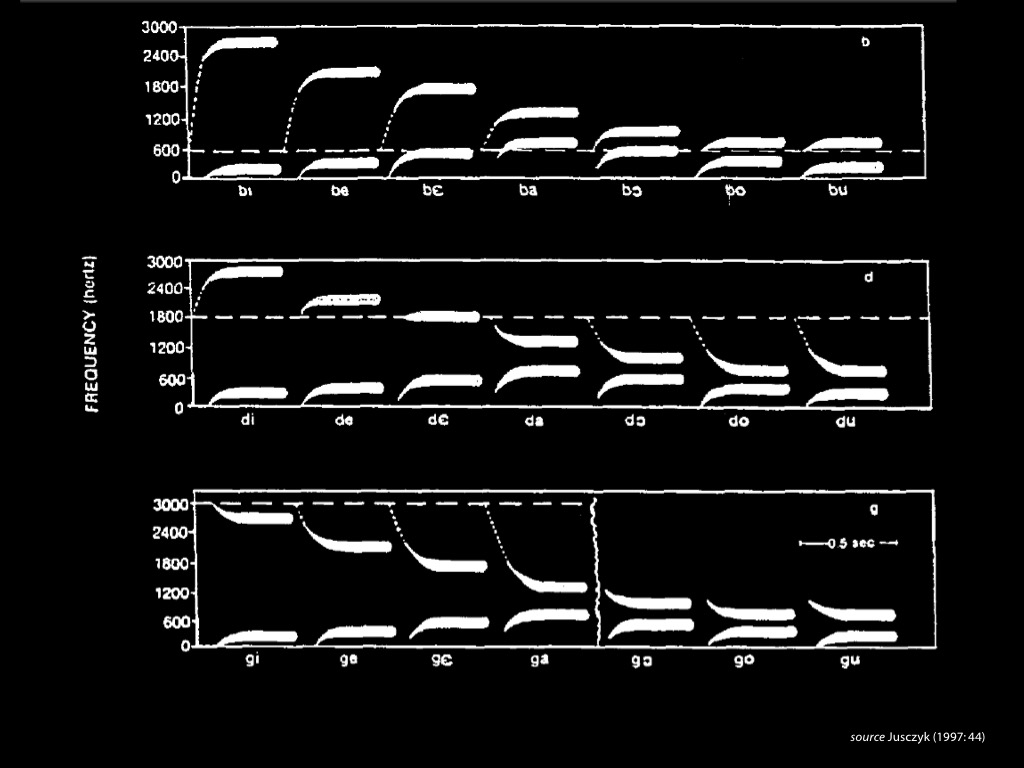
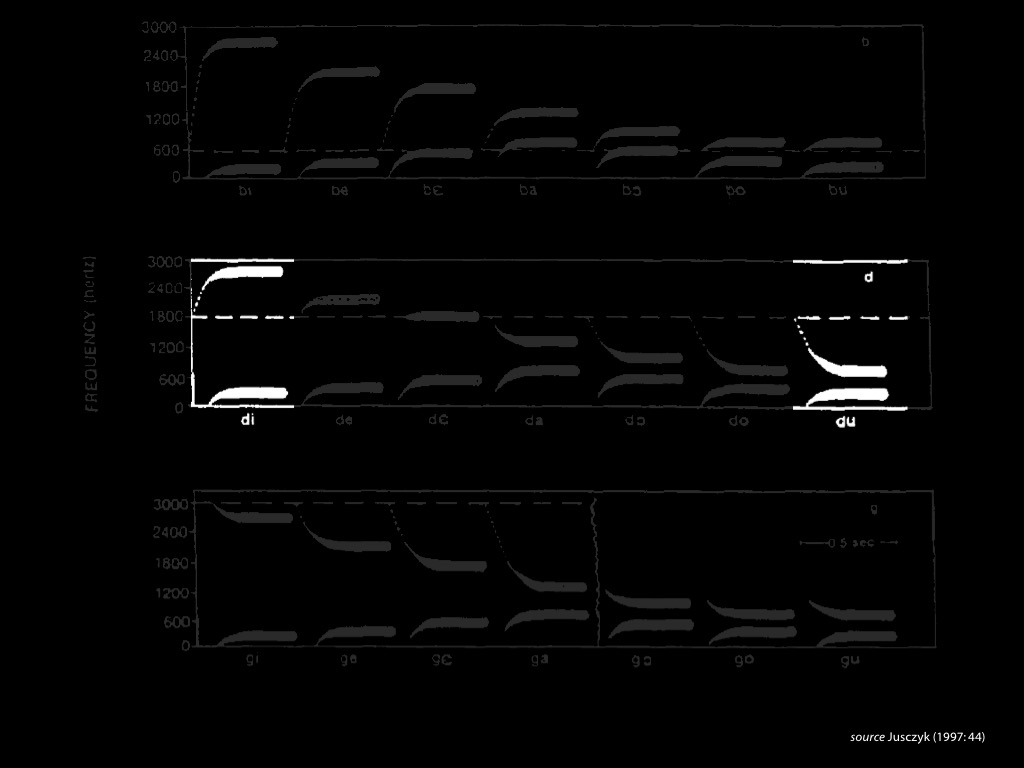

Distinct questions ...
| change phonetic context | change dialect, rate, ... | |
| Same sound? | ✗ | ✓ |
| Same phonetic gesture? | ✓ | ✗ |
... but are there distinct systems?
1. computational description
-- What is the thing for and how does it achieve this?
2. representations and algorithms
-- How are the inputs and outputs represented, and how is the transformation accomplished?
3. hardware implementation
-- How are the representations and algorithms physically realised?
Marr (1992, 22ff)
Distinct questions computational descriptions ...
| change phonetic context | change dialect, rate, ... | |
| Same sound? | ✗ | ✓ |
| Same phonetic gesture? | ✓ | ✗ |
... but are there distinct systems?
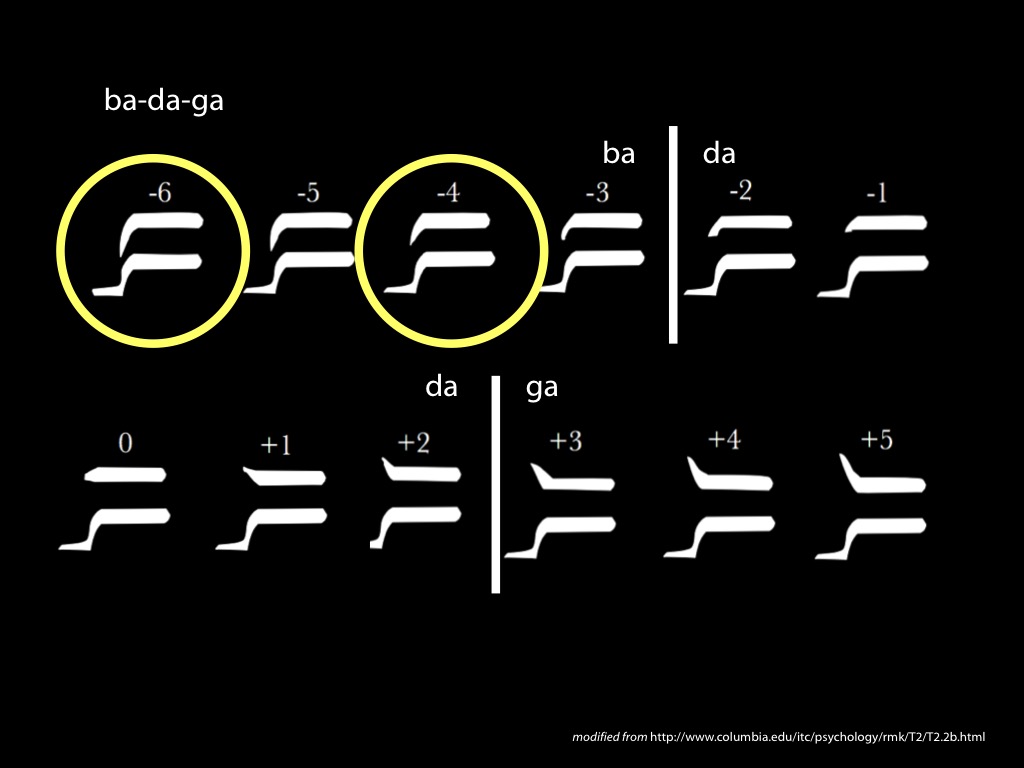
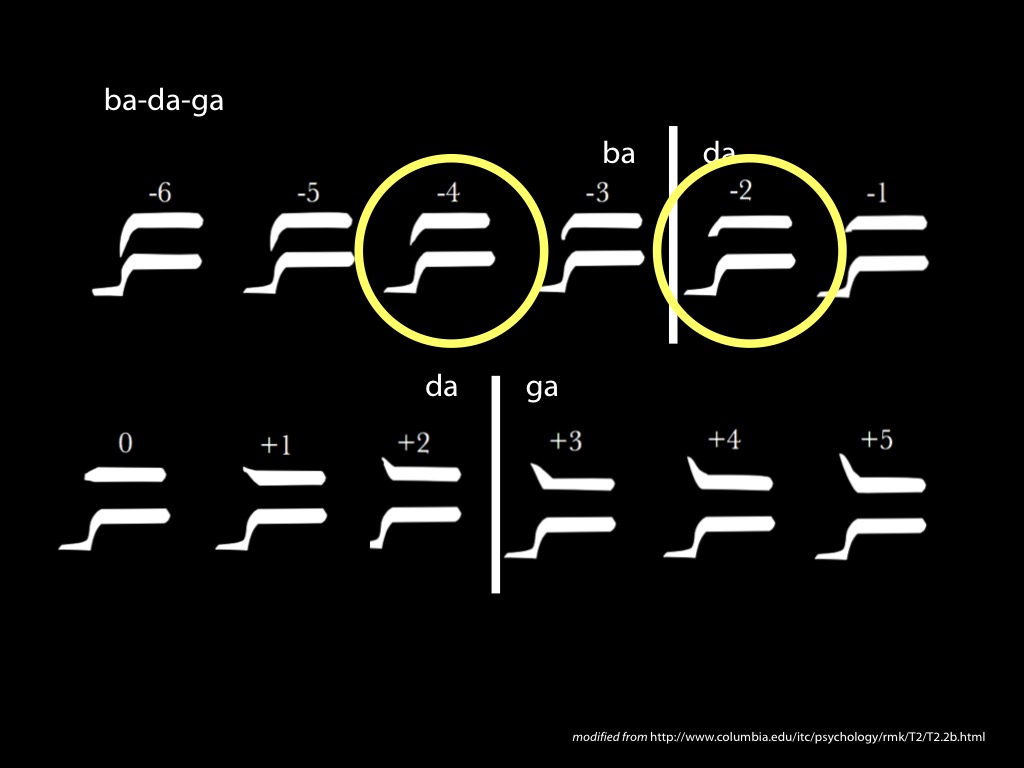
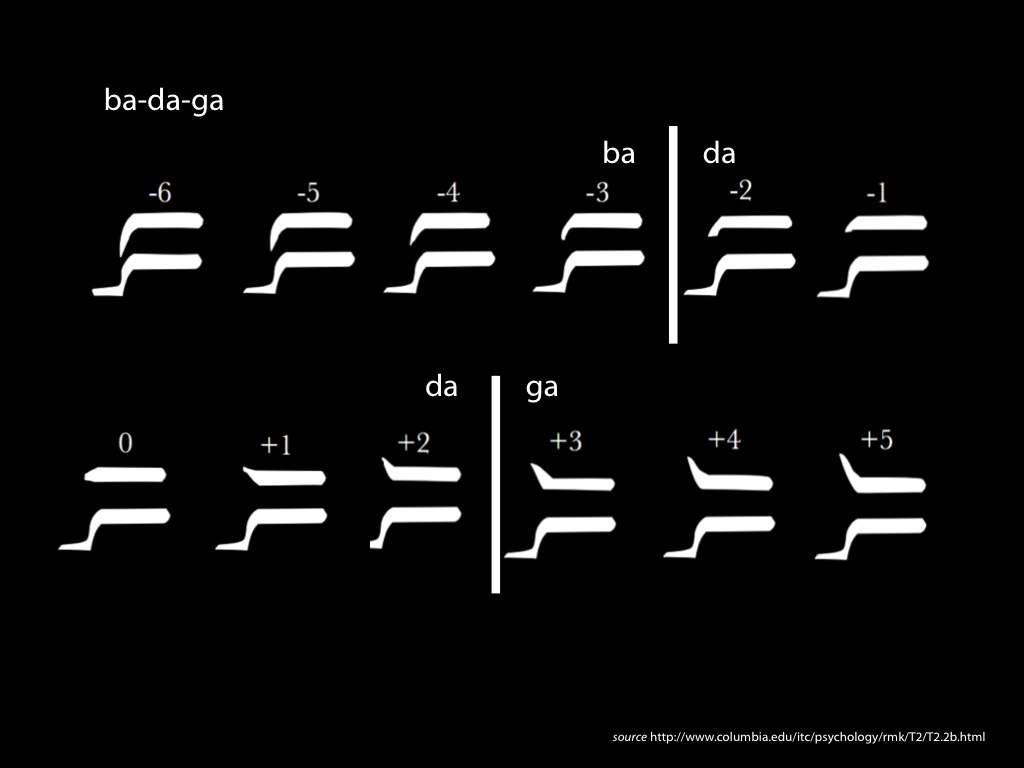
The Argument From ‘Jein’
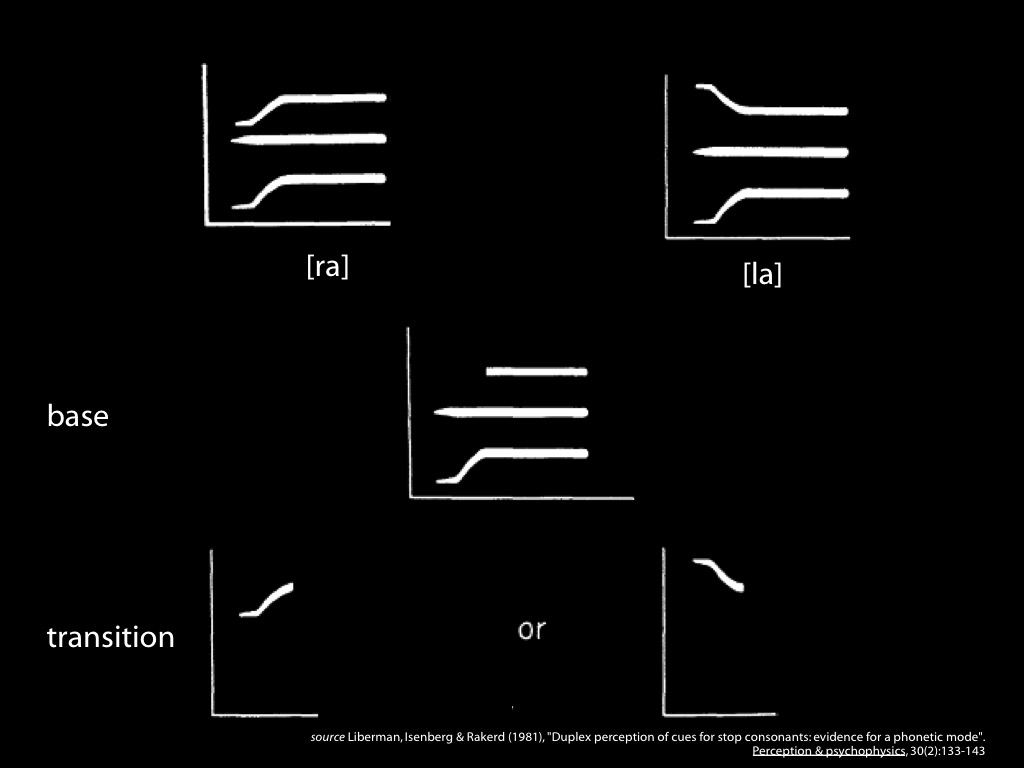
The Argument From ‘Jein’
Multiple systems make possible what a single system rules out: incompatible responses to a single stimulus.
The Timing Objection
A single system makes incompatible responses sequentially.
The Objection from ‘Meh’
A single system puts roughly equal weight on two answers.

system ?!

physical cognition
phonological awareness
physical cognition
speech perception
auditory perception
case study 2
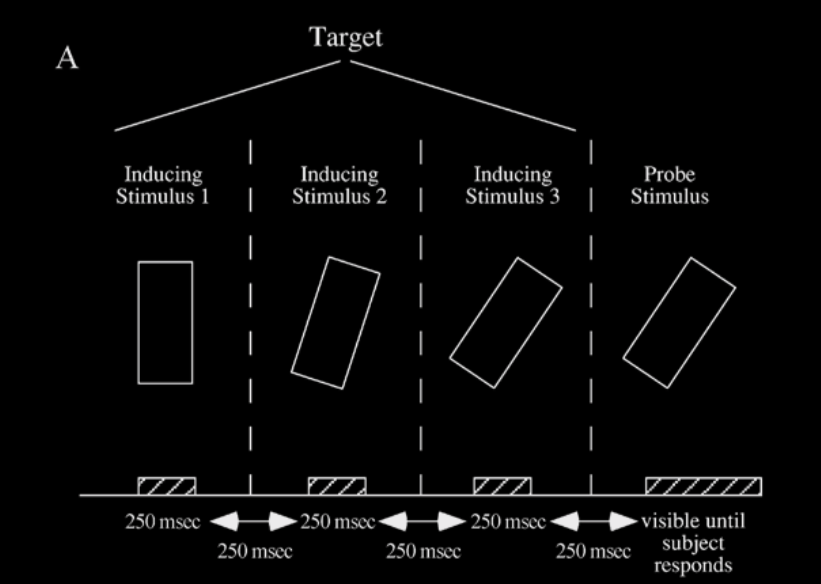
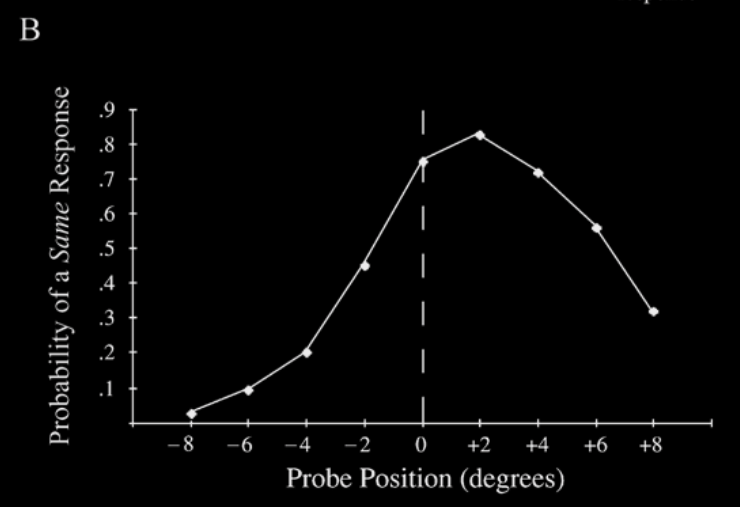
representational momentum

Hubbard 2005, figure 1a; redrawn from Freyd and Finke 1984, figure 1
Hubbard 2005, figure 1b; drawn from Freyd and Finke 1984, table 1
Is the system underpinning representational momentum also what underpins explicit verbal predictions about objects’ trajectories?
speed vs accuracy
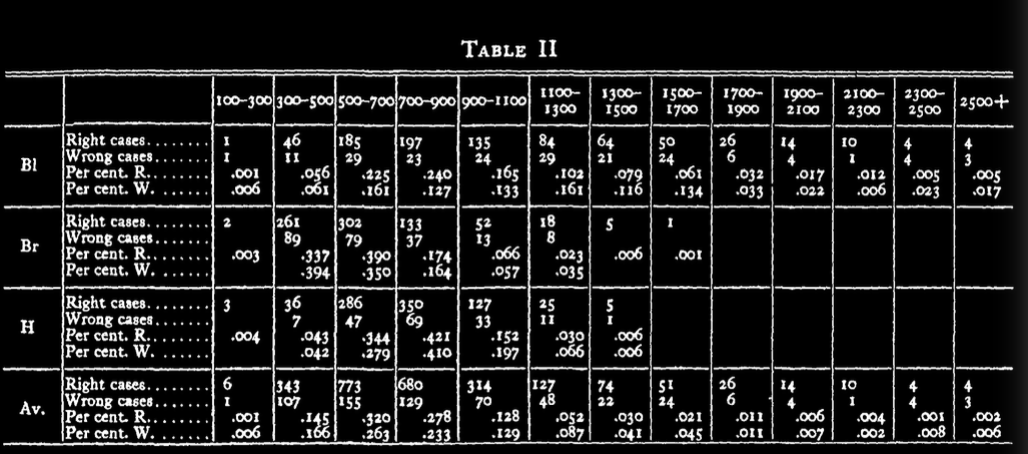
Henmon (1911, table 2)
Is the system underpinning representational momentum also what underpins explicit verbal predictions about objects’ trajectories?
How could we tell?
1. computational description
-- What is the thing for and how does it achieve this?
2. representations and algorithms
-- How are the inputs and outputs represented, and how is the transformation accomplished?
3. hardware implementation
-- How are the representations and algorithms physically realised?
Marr (1992, 22ff)
‘To extrapolate objects’ motion on the basis of [e.g. Newtonian] physical principles, one should have assessed and evaluated the presence and magnitude of such imperceptible forces as friction and air resistance ... This would require a time-consuming analysis that is not always possible.
‘In order to have a survival advantage, the process of extrapolation should be fast and effortless, without much conscious deliberation.
‘Impetus theory allows us to extrapolate objects’ motion quickly and without large demands on attentional resources.’
Kozhevnikov and Heggarty (2001, p. 450)
How to get from computational description to prediction?
Signature limits!

Kozhevnikov & Hegarty (2001, figure 1)
simplified from Kozhevnikov & Hegarty (2001)
simplified from Kozhevnikov & Hegarty (2001)
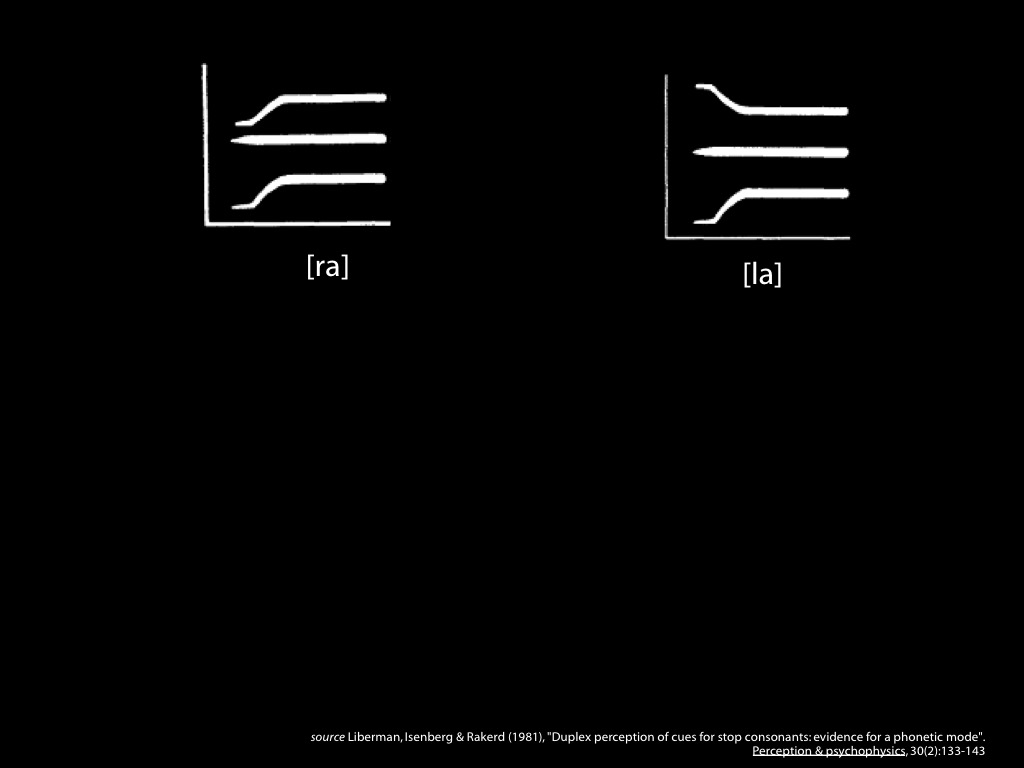
From speech perception (Liberman et al)
Multiple systems make possible what a single system rule out: incompatible responses to a single stimulus
From representational momentum (Kozhevnikov & Heggarty):
1. speed-vs-accuracy trade offs
motivate conjectures about
2. computational descriptions
which entail
3. signature limits
that generate predictions.
mental state ascription
From speech perception (Liberman et al)
Multiple systems make possible what a single system rule out: incompatible responses to a single stimulus
From representational momentum (Kozhevnikov & Heggarty):
1. speed-vs-accuracy trade offs
motivate conjectures about
2. computational descriptions
which entail
3. signature limits
that generate predictions.
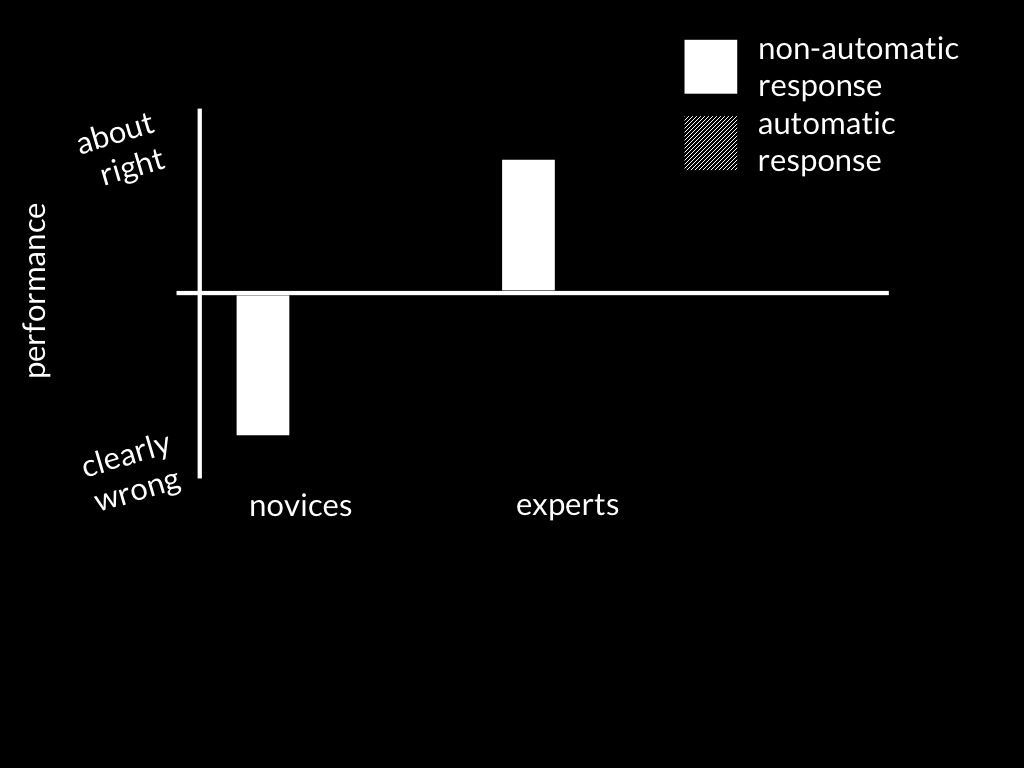
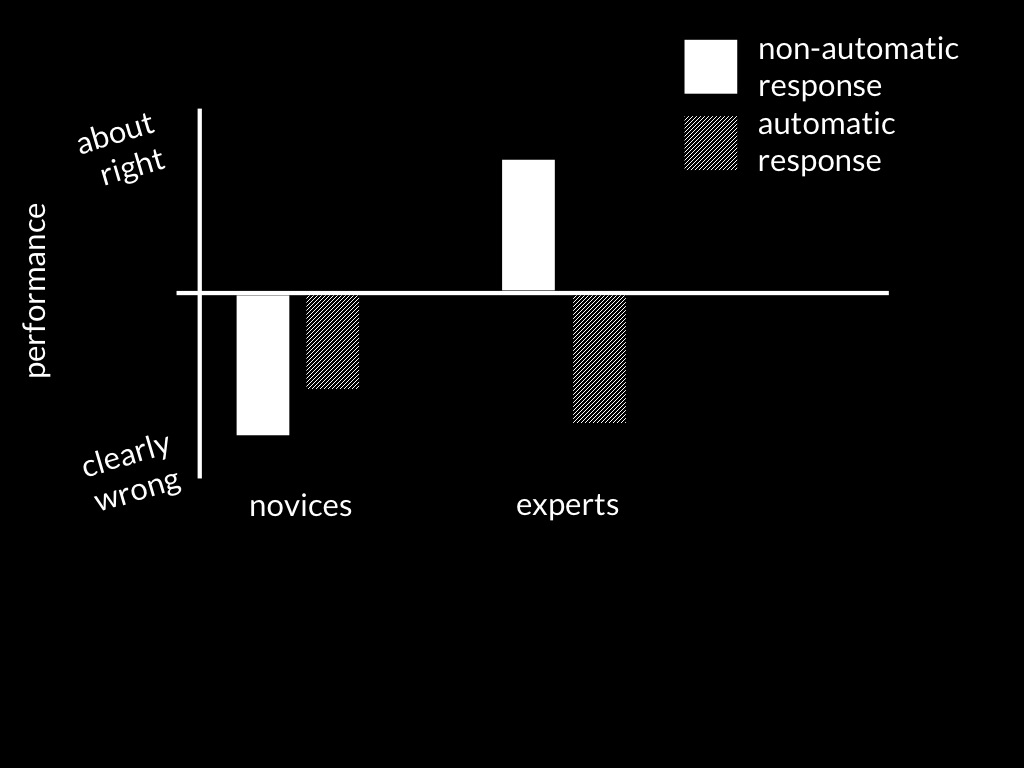
belief-tracking is sometimes but not always automatic
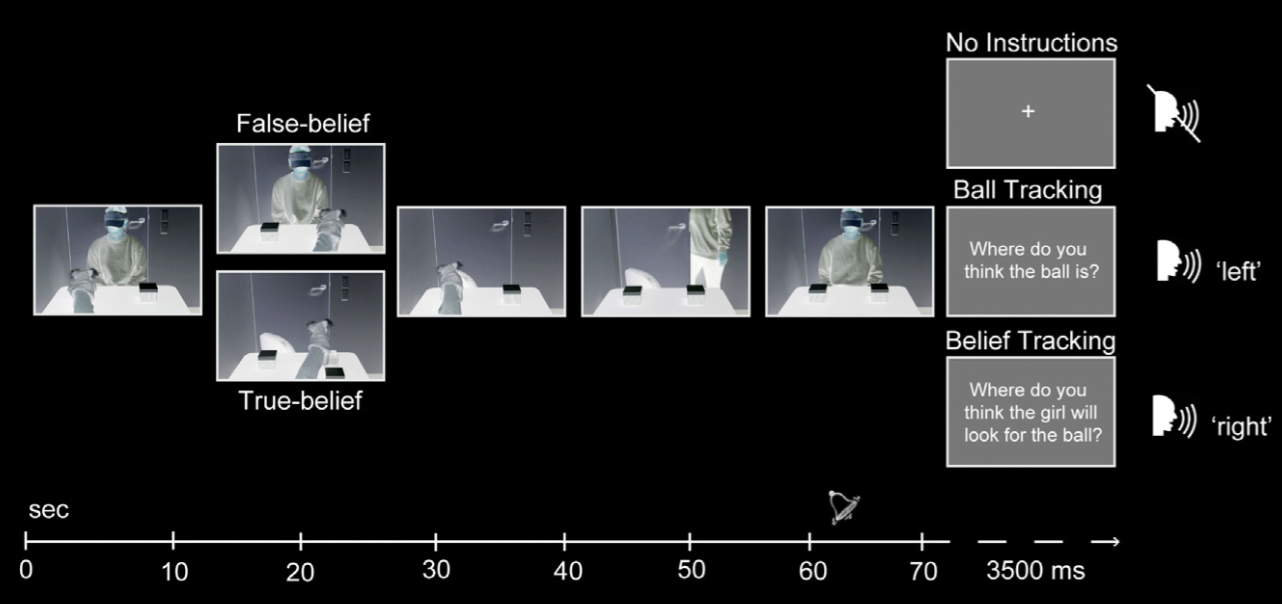
Schneider et al (2014, figure 1)
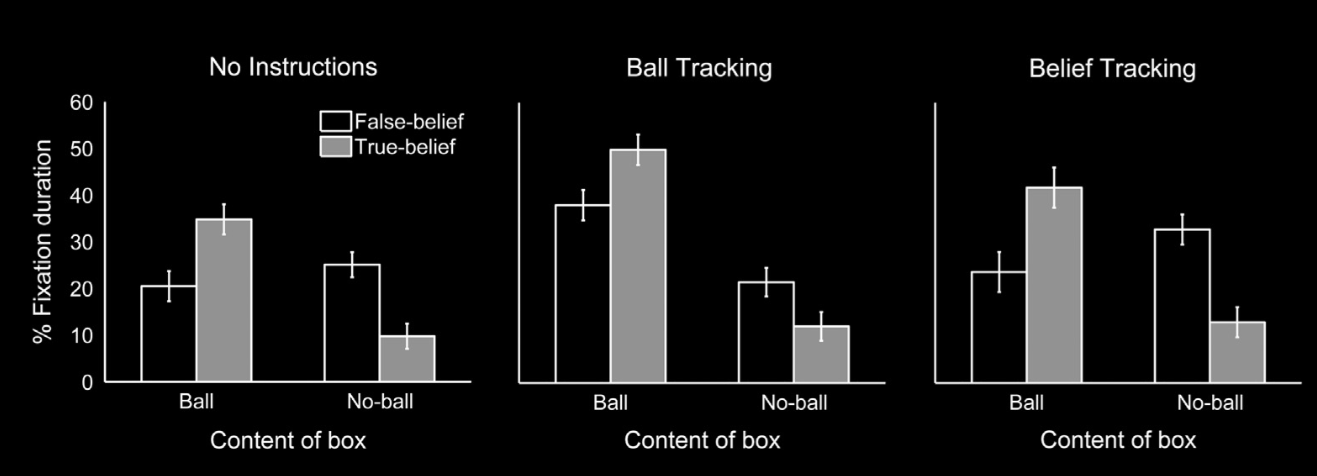
Schneider et al (2014, figure 3)
From speech perception (Liberman et al)
Multiple systems make possible what a single system rule out: incompatible responses to a single stimulus
From representational momentum (Kozhevnikov & Heggarty):
1. speed-vs-accuracy trade offs
motivate conjectures about
2. computational descriptions
which entail
3. signature limits
that generate predictions.
the
dogma
of mindreading



From speech perception (Liberman et al)
Multiple systems make possible what a single system rule out: incompatible responses to a single stimulus
From representational momentum (Kozhevnikov & Heggarty):
1. speed-vs-accuracy trade offs
motivate conjectures about
2. computational descriptions
which entail
3. signature limits
that generate predictions.
 |  |
From speech perception (Liberman et al)
Multiple systems make possible what a single system rule out: incompatible responses to a single stimulus
From representational momentum (Kozhevnikov & Heggarty):
1. speed-vs-accuracy trade offs
motivate conjectures about
2. computational descriptions
which entail
3. signature limits
that generate predictions.
Hypothesis:
Some automatic belief-tracking systems rely on minimal models of the mental.
Prediction:
Automatic belief-tracking is subject to the signature limits of minimal models.





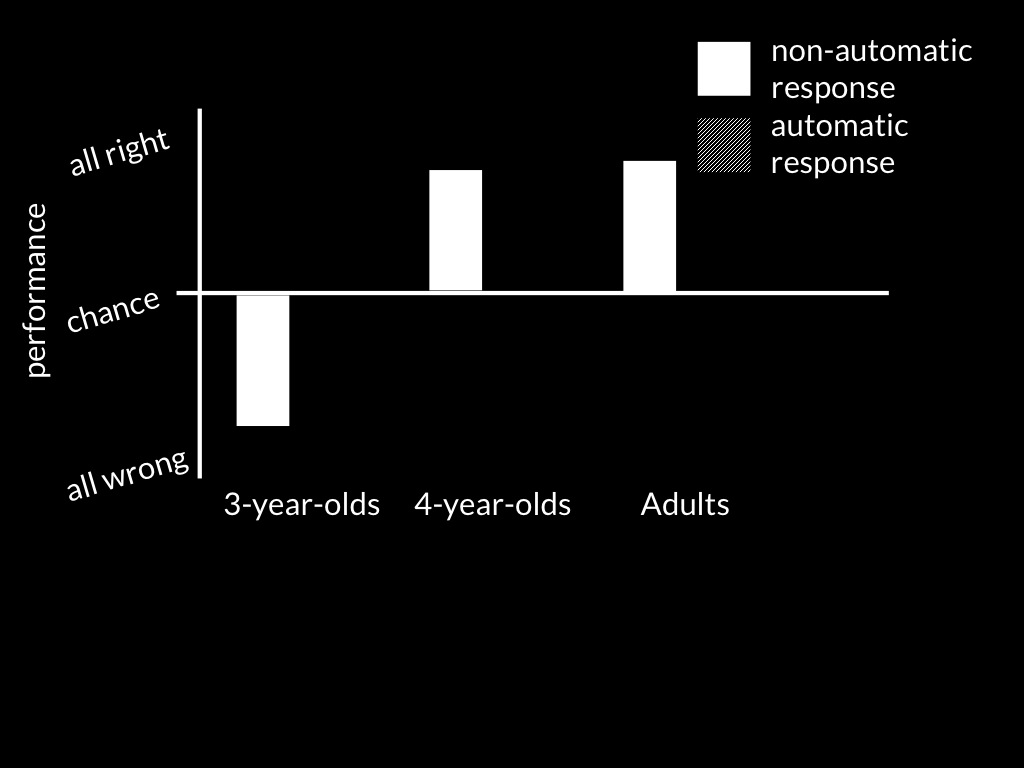
False-Belief:Identity task
adapted from Low & Watts (2013); Low et al (2014); Wang et al (2015)
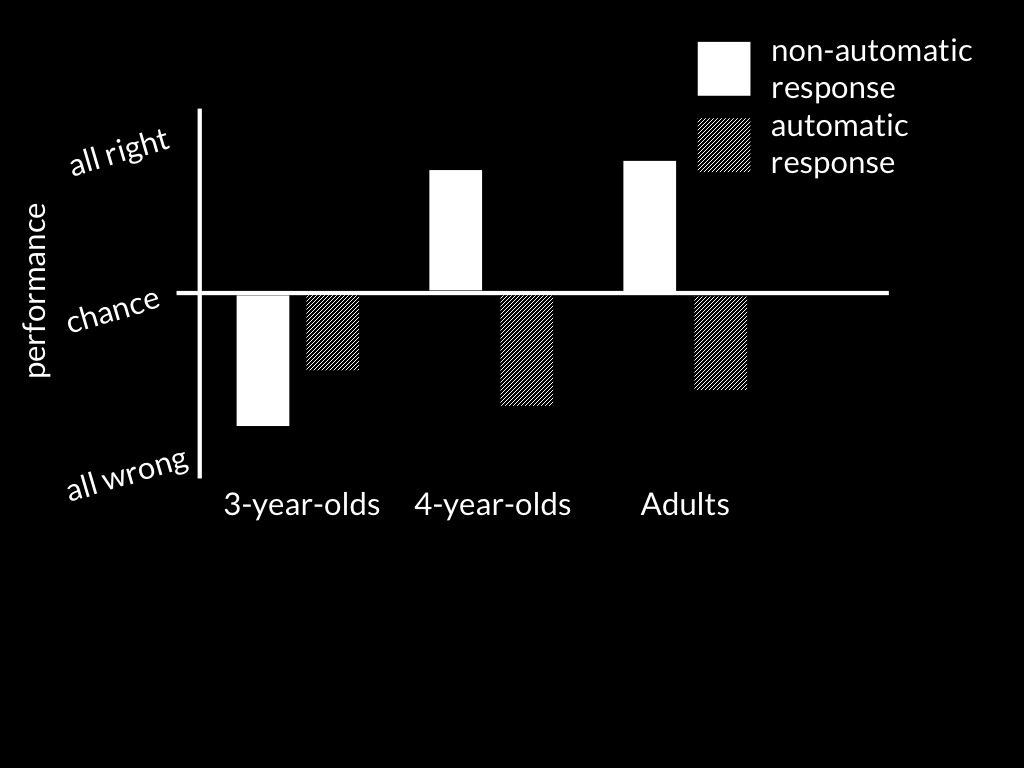
False-Belief:Identity task
adapted from Low & Watts (2013); Low et al (2014); Wang et al (2015)
reidentifying systems:
same signature limit -> same system

False-Belief:Identity task
adapted from Low & Watts (2013); Low et al (2014); Wang et al (2015)

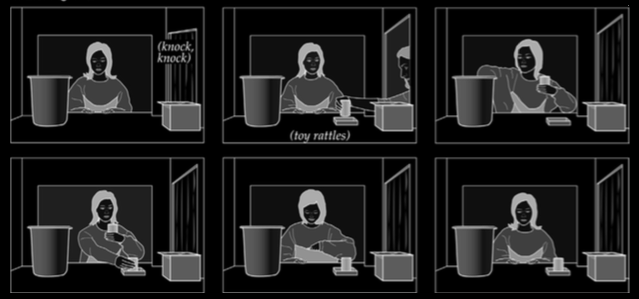
Scott et al (2015, figure 2b)
From speech perception (Liberman et al)
Multiple systems make possible what a single system rule out: incompatible responses to a single stimulus
From representational momentum (Kozhevnikov & Heggarty):
1. speed-vs-accuracy trade offs
motivate conjectures about
2. computational descriptions
which entail
3. signature limits
that generate predictions.

conclusion
‘the theoretical arguments offered [...] are [...] unconvincing, and [...] the data can be explained in other terms’
Carruthers (2015)
The system underpinning automatic belief tracking in adults
is not what generally underpins explicit verbal judgements;
and it relies on minimal theory of mind.
The same system underpins
some of infants’ belief-tracking abilities; or even
all of infants’ belief-tracking abilities.